Tack question in LOG

Utilize this walkthrough to tack question in LOG in a snap

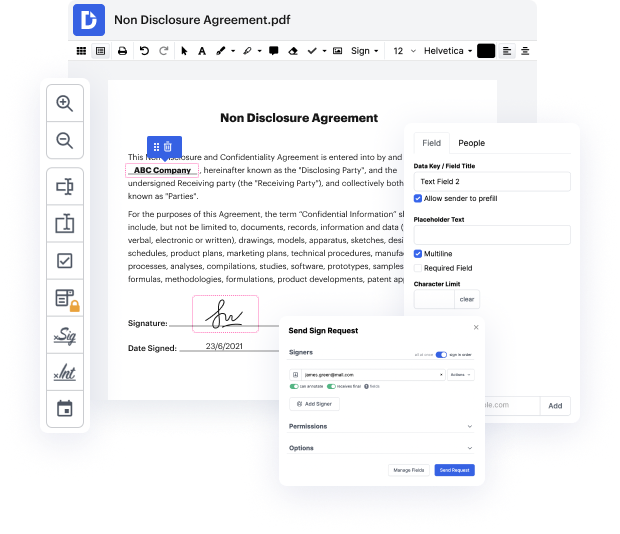
LOG may not always be the simplest with which to work. Even though many editing tools are out there, not all offer a easy solution. We designed DocHub to make editing easy, no matter the form format. With DocHub, you can quickly and effortlessly tack question in LOG. Additionally, DocHub delivers an array of other functionality including form creation, automation and management, field-compliant eSignature tools, and integrations.

DocHub also helps you save time by producing form templates from paperwork that you utilize regularly. Additionally, you can take advantage of our a wide range of integrations that enable you to connect our editor to your most used programs effortlessly. Such a solution makes it quick and easy to work with your files without any delays.

To tack question in LOG, follow these steps:

Hit Log In or create a free account.
When directed to your Dashboard, hit the Add New button and choose how you want to add your form.
Use our sophisticated tools that will let you enhance your document's text and design.
Choose the option to tack question in LOG from the toolbar and use it on form.
Review your text once again to make sure it has no errors or typos.
Hit DONE to complete editing form.

DocHub is a helpful feature for personal and corporate use. Not only does it offer a extensive collection of tools for form generation and editing, and eSignature implementation, but it also has an array of tools that prove useful for producing complex and straightforward workflows. Anything added to our editor is kept safe in accordance with leading field standards that shield users' information.

Make DocHub your go-to option and streamline your form-driven workflows effortlessly!

PDF editing simplified with DocHub

Seamless PDF editing

Editing a PDF is as simple as working in a Word document. You can add text, drawings, highlights, and redact or annotate your document without affecting its quality. No rasterized text or removed fields. Use an online PDF editor to get your perfect document in minutes.

Smooth teamwork

Collaborate on documents with your team using a desktop or mobile device. Let others view, edit, comment on, and sign your documents online. You can also make your form public and share its URL anywhere.

Automatic saving

Every change you make in a document is automatically saved to the cloud and synchronized across all devices in real-time. No need to send new versions of a document or worry about losing information.

Google integrations

DocHub integrates with Google Workspace so you can import, edit, and sign your documents directly from your Gmail, Google Drive, and Dropbox. When finished, export documents to Google Drive or import your Google Address Book and share the document with your contacts.

Powerful PDF tools on your mobile device

Keep your work flowing even when you're away from your computer. DocHub works on mobile just as easily as it does on desktop. Edit, annotate, and sign documents from the convenience of your smartphone or tablet. No need to install the app.

Secure document sharing and storage

Instantly share, email, and fax documents in a secure and compliant way. Set a password, place your documents in encrypted folders, and enable recipient authentication to control who accesses your documents. When completed, keep your documents secure in the cloud.

Drive efficiency with the DocHub add-on for Google Workspace

Access documents and edit, sign, and share them straight from your favorite Google Apps.

Install now

How to tack question in LOG

4.6 out of 5

8 votes

consider this complex logarithmic equation what would you do to solve it feel free to pause the video if you want to give this problem a shot go ahead and try now before we tackle this problem there are some things we need to review that is the ability to convert a logarithmic expression into an exponential one for instance consider this formula log base a of b is equal to c we can convert it into its exponential form by following this process a raised to the c is equal to b so letamp;#39;s say we have log base 4 of 64 is equal to 3. we could say that 4 raised to the third power is equal to 64. so weamp;#39;re going to do a lot of that in this particular problem so letamp;#39;s begin the first thing we could do is say three raised to the second power is equal to everything else here everything in the red brackets so letamp;#39;s write that 3 squared is equal to 7 plus log base 4 of 13 plus log base 2 of x plus 4. now three squared is equal to nine and so we have nine is equal to se

Related features

Replace light in the Clinical Trial Agreement Template

Replace light in the Sponsorship Agreement Template

Replace light in the Rent to Own Agreement Template

Replace light in the Car Purchase Agreement Template

Replace light in the Web Hosting Agreement Template

Replace light in the Endorsement Agreement Template

Replace light in the SEO Agreement Template

Replace light in the Collaboration Agreement Template

Got questions?

Below are some common questions from our customers that may provide you with the answer you're looking for. If you can't find an answer to your question, please don't hesitate to reach out to us.

Contact us

What are the 5 log rules?

What are the 7 Log Rules? Rule NameLog Rule Product Rule logb mn = logb m + logb n Quotient Rule logb m/n = logb m - logb n Power Rule of Logarithm logb mn = n logb m Change of Base Rule logb a = (log a) / ( log b)3 more rows

What are the three basic log rules?

Comparison of Exponent law and Logarithm law Properties/RulesExponentsLogarithms Product Rule xp.xq = xp+q loga(mn) = logam + logan Quotient Rule xp/xq = xp-q loga(m/n) = logam logan Power Rule (xp)q = xpq logamn = n logam

What are the three log rules?

Product Law: log⁡b(xy) = log⁡b⁡(x) + logb(y) Quotient Law: log⁡b(xy)=log⁡b(x)log⁡b(y) Power Law: log⁡b(xk)=klog⁡b(x)

What are the 3 types of logarithms?

Particular bases Base bName for logb xOther notations 2 binary logarithm ld x, log x, lg x, log2 x e natural logarithm log x, loge x 10 common logarithm log x, log10 x b logarithm to base b

How to do log questions?

0:01 25:26 Now you might already see the answer but im going to go ahead and solve. It. Now we know that 2 toMoreNow you might already see the answer but im going to go ahead and solve. It. Now we know that 2 to the 4th power is 16..

What are the 7 properties of logarithms?

What are All Properties of Logarithms? log 1 = 0. logₐ a = 1. log ab = log a + log b. log a/b = log a - log b. log am = m log a. logba = (log a)/(log b) alogₐ x = x.

What are the three 3 components of a logarithmic equation?

Just as an exponential function has three parts, a logarithm has three parts as well: a base, a power and an answer (also called exponent).

What are the 3 logarithmic properties?

In words, the first three can be remembered as: The log of a product is equal to the sum of the logs of the factors. The log of a quotient is equal to the difference between the logs of the numerator and demoninator. The log of a power is equal to the power times the log of the base.

See why our customers choose DocHub

Great solution for PDF docs with very little pre-knowledge required.

"Simplicity, familiarity with the menu and user-friendly. It's easy to navigate, make changes and edit whatever you may need. Because it's used alongside Google, the document is always saved, so you don't have to worry about it."

Pam Driscoll F
Teacher

A Valuable Document Signer for Small Businesses.

"I love that DocHub is incredibly affordable and customizable. It truly does everything I need it to do, without a large price tag like some of its more well known competitors. I am able to send secure documents directly to me clients emails and via in real time when they are viewing and making alterations to a document."

Jiovany A
Small-Business

I can create refillable copies for the templates that I select and then I can publish those.

"I like to work and organize my work in the appropriate way to meet and even exceed the demands that are made daily in the office, so I enjoy working with PDF files, I think they are more professional and versatile, they allow..."

Victoria G
Small-Business

be ready to get more

Edit and sign PDFfor free

Get started now