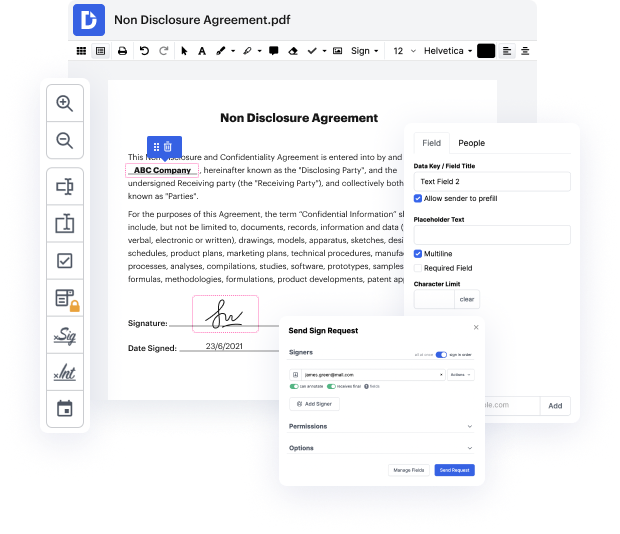
No matter how complex and challenging to change your documents are, DocHub offers a straightforward way to modify them. You can modify any part in your SDW with no effort. Whether you need to tweak a single element or the entire document, you can entrust this task to our powerful tool for fast and quality results.
Moreover, it makes certain that the final form is always ready to use so that you can get on with your tasks without any slowdowns. Our all-purpose group of tools also features pro productivity tools and a collection of templates, allowing you to take full advantage of your workflows without losing time on routine operations. Additionally, you can access your documents from any device and incorporate DocHub with other solutions.
DocHub can handle any of your document management operations. With a great deal of tools, you can create and export paperwork however you want. Everything you export to DocHub’s editor will be saved safely for as long as you need, with strict security and data protection frameworks in place.
Experiment with DocHub today and make handling your files easier!
weamp;#39;ve seen two different matrix decompositions the singular value decomposition and the QR decomposition what I want to do in this video is demonstrating how both of these are useful for solving systems of equations the fundamental problem is linear algebra is to solve the problem ax equals B you have a fixed matrix a and a fix matrix B and you want to find the x solve this linear system of equations thatamp;#39;s where it all begins now of course youamp;#39;d say well perhaps a has an inverse we can apply your operations to find a inverse and then x is a inverse B thatamp;#39;s certainly true as far as it goes but perhaps a inverses I exist but we still on two solutions say using some reduced row echelon form of a and their operations a pseudo inverse on their hand it turns out this operation of Denver operations is not exactly numerically stable if you have small errors an A or B they can accumulate to this process of of manipulation and division of the different rows and