Disadvantages are present in every solution for editing every file type, and even though you can find a lot of tools on the market, not all of them will suit your particular needs. DocHub makes it much simpler than ever to make and modify, and handle papers - and not just in PDF format.
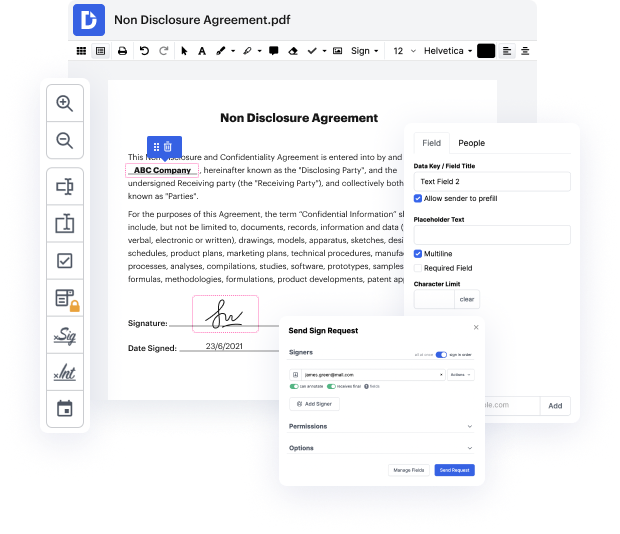
Every time you need to easily strike out margin in EZW, DocHub has got you covered. You can quickly modify form elements such as text and pictures, and layout. Personalize, organize, and encrypt documents, build eSignature workflows, make fillable documents for smooth data collection, and more. Our templates option allows you to create templates based on papers with which you frequently work.
Moreover, you can stay connected to your go-to productivity tools and CRM platforms while dealing with your documents.
One of the most incredible things about leveraging DocHub is the option to handle form activities of any difficulty, regardless of whether you need a swift modify or more complex editing. It includes an all-in-one form editor, website document builder, and workflow-centered tools. Moreover, you can rest assured that your papers will be legally binding and abide by all protection frameworks.
Shave some time off your projects with DocHub's features that make managing documents easy.
hello everyone in this video we are going to consider what happens when we place a Charged particle in other words a point charge outside a conducting sphere so weamp;#39;ve got a diagram of the setup up at the top left of the screen um weamp;#39;ve got our sphere with radius R Iamp;#39;ve put this x here representing the center of the sphere which is also the origin of our coordinate system so weamp;#39;re placing the sphere at the origin of our coordinate system and our charged particle is over here it has a charge of capital Q itamp;#39;s on the x-axis and so it has coordinates of d 0 0 and so D is the the distance along the x-axis from the center of the sphere so the way in which weamp;#39;re going to approach this is to use the method of images which in turn relies on the uniqueness theorem for poissonamp;#39;s equation um I have done a video on that in the past if youamp;#39;re not familiar with that but the basic idea is that in electrostatics the surface of a conductor