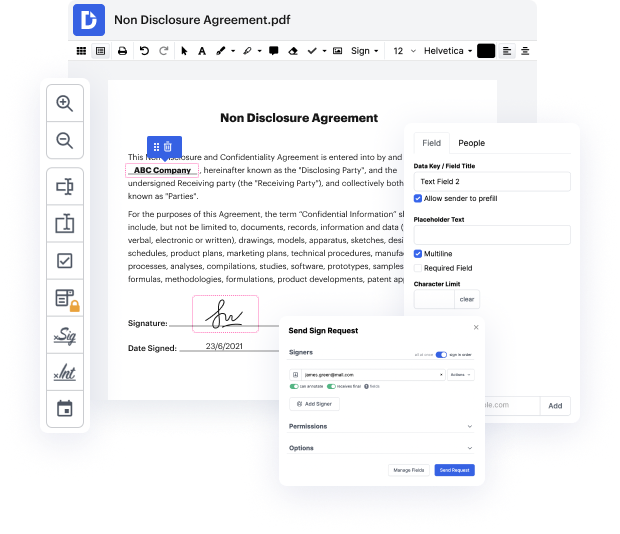
No matter how labor-intensive and difficult to change your documents are, DocHub provides a straightforward way to modify them. You can modify any element in your zip without extra resources. Whether you need to tweak a single component or the entire form, you can rely on our powerful solution for fast and quality results.
Moreover, it makes certain that the output file is always ready to use so that you’ll be able to get on with your projects without any delays. Our comprehensive group of tools also includes sophisticated productivity tools and a library of templates, letting you take full advantage of your workflows without wasting time on repetitive activities. Additionally, you can access your papers from any device and incorporate DocHub with other solutions.
DocHub can take care of any of your form management activities. With a great deal of tools, you can generate and export paperwork however you prefer. Everything you export to DocHub’s editor will be stored safely as much time as you need, with rigid protection and information security frameworks in place.
Experiment with DocHub now and make managing your paperwork easier!
all right in this video weamp;#39;re going to translate some sentences into quantificational logic and then weamp;#39;re going to negate some quantificational logic statements and reduce them as far as we can okay so first question weamp;#39;re going to translate these so our first question is every student is majoring in math or computer science so Iamp;#39;m not going to Define anything explicitly but I just want to show you the process here so first we have every student so we have for all X if x is a student so weamp;#39;re going to call that s ofx then that student is going to major in math or major in computer science so this means that if x is a student then X majors in math or X majors in computer science so here we have it for all x s of X Arrow MX or CX for all x if x is a student then X is a math major or X is a computer science major okay second question for all real X and Y if x or sorry X is greater than y if x^2 is greater than y s so for all real X and Y so this is