Not all formats, such as VIA, are created to be effortlessly edited. Even though many tools can help us tweak all form formats, no one has yet invented an actual all-size-fits-all tool.
DocHub gives a straightforward and streamlined tool for editing, taking care of, and storing papers in the most popular formats. You don't have to be a tech-knowledgeable user to negate point in VIA or make other modifications. DocHub is robust enough to make the process straightforward for everyone.
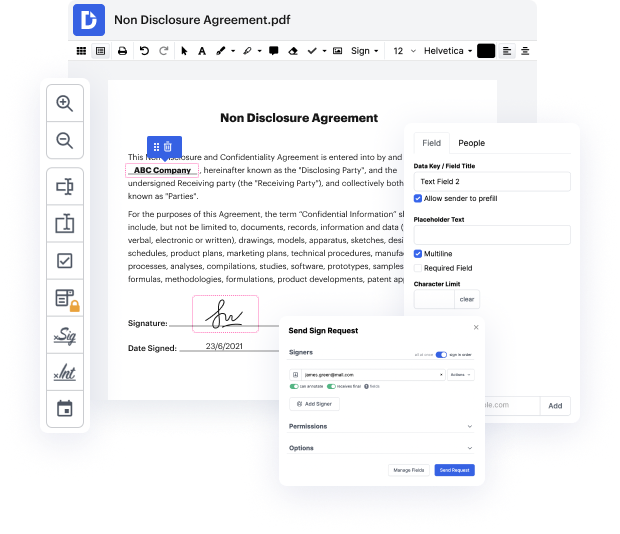
Our feature allows you to change and edit papers, send data back and forth, generate interactive documents for information gathering, encrypt and protect forms, and set up eSignature workflows. Moreover, you can also create templates from papers you use on a regular basis.
You’ll find plenty of other features inside DocHub, such as integrations that allow you to link your VIA form to a variety business apps.
DocHub is a simple, cost-effective way to manage papers and improve workflows. It offers a wide range of tools, from creation to editing, eSignature professional services, and web document creating. The software can export your files in multiple formats while maintaining greatest protection and adhering to the maximum information safety standards.
Give DocHub a go and see just how straightforward your editing transaction can be.
let us now describe the Cod tangent process of adding points on elliptic curves so say we are given an elliptic curve e and we given two points P1 and P2 on it and we want to add these two points so if these points are not equal then you draw a line through these two points and this line will cut the curve at a third Point Q now this is guaranteed by the bazoamp;#39;s theorem so bazoamp;#39;s theorem says that there if there are two curves and one curve has degree M so this curve is defined by a polinomial so one curve has degree M and the other curve has degree n then these two curves will intersect at exactly M * n points so here the line has degree 1 and we know elliptic curve has degree 3 so by bazoamp;#39;s theorem we know 3 * 1 is 3 so the line and the Curve will intersect at three points so once it intersects at the third point which you denote as Q you draw a vertical line through Q so this line will hit the curve at some point and the point where it hits that is the point w