Negate clause in EZW

Use this walkthrough to negate clause in EZW in a snap

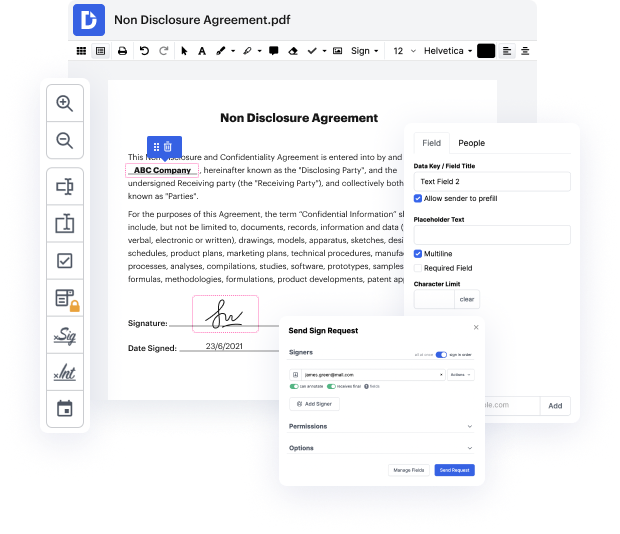
EZW may not always be the easiest with which to work. Even though many editing tools are out there, not all offer a straightforward tool. We created DocHub to make editing easy, no matter the document format. With DocHub, you can quickly and effortlessly negate clause in EZW. Additionally, DocHub offers an array of other functionality such as form creation, automation and management, field-compliant eSignature tools, and integrations.

DocHub also helps you save time by producing form templates from paperwork that you use regularly. Additionally, you can make the most of our a wide range of integrations that allow you to connect our editor to your most utilized programs easily. Such a tool makes it quick and easy to work with your files without any slowdowns.

To negate clause in EZW, follow these steps:

Hit Sign In or create a free account.
When forwarded to your Dashboard, click the Add New button and select how you want to import your document.
Use our advanced capabilities that will let you enhance your document's content and layout.
Pick the ability to negate clause in EZW from the toolbar and apply it to form.
Review your content once more to ensure it has no errors or typos.
Hit DONE to finish working on your form.

DocHub is a handy tool for personal and corporate use. Not only does it offer a comprehensive set of capabilities for form creation and editing, and eSignature implementation, but it also has an array of tools that come in handy for developing multi-level and streamlined workflows. Anything uploaded to our editor is saved secure in accordance with major field criteria that safeguard users' data.

Make DocHub your go-to choice and simplify your form-centered workflows easily!

PDF editing simplified with DocHub

Seamless PDF editing

Editing a PDF is as simple as working in a Word document. You can add text, drawings, highlights, and redact or annotate your document without affecting its quality. No rasterized text or removed fields. Use an online PDF editor to get your perfect document in minutes.

Smooth teamwork

Collaborate on documents with your team using a desktop or mobile device. Let others view, edit, comment on, and sign your documents online. You can also make your form public and share its URL anywhere.

Automatic saving

Every change you make in a document is automatically saved to the cloud and synchronized across all devices in real-time. No need to send new versions of a document or worry about losing information.

Google integrations

DocHub integrates with Google Workspace so you can import, edit, and sign your documents directly from your Gmail, Google Drive, and Dropbox. When finished, export documents to Google Drive or import your Google Address Book and share the document with your contacts.

Powerful PDF tools on your mobile device

Keep your work flowing even when you're away from your computer. DocHub works on mobile just as easily as it does on desktop. Edit, annotate, and sign documents from the convenience of your smartphone or tablet. No need to install the app.

Secure document sharing and storage

Instantly share, email, and fax documents in a secure and compliant way. Set a password, place your documents in encrypted folders, and enable recipient authentication to control who accesses your documents. When completed, keep your documents secure in the cloud.

Drive efficiency with the DocHub add-on for Google Workspace

Access documents and edit, sign, and share them straight from your favorite Google Apps.

Install now

How to negate clause in EZW

4.8 out of 5

61 votes

so weamp;#39;ve seen now how the magic equation for tricolor ability gives us a computational tool for determining whether a given knot has a tri coloration and if it does have a tri coloration how many tri colorations does it have this seems like it could be a really powerful way to distinguish between different types of knots the limitation that it has though is that it only uses three colors and tri coloration might not be enough to distinguish between knots in general we may need to use four colors or five or seven or 12 or 18 or 212 right what weamp;#39;d like to do is push the envelope a little bit and figure out how to go from a tri color ability condition into aka color ability condition if I have K colors to work with how do I come up with a new magic equation which I can then use to build a square system of linear equations that I can then use modular arithmetic to determine whether or not solutions exist for that K coloration system of equations this is going to be ultimat

Related features

Link style in the Website Analytics Proposal Template

Link style in the HTML 5 App & Website Proposal

Link style in the Facebook Marketing Proposal Template

Link style in the Social Media Optimization Proposal Template

Link style in the Affiliate Marketing Proposal Template

Link style in the Website Strategy Audit Proposal Template

Link style in the Event Marketing Proposal Template

Link style in the Responsive Design Proposal Template

Got questions?

Below are some common questions from our customers that may provide you with the answer you're looking for. If you can't find an answer to your question, please don't hesitate to reach out to us.

Contact us

What is EZW in Matlab?

Image compression algorithms based on Discrete Wavelet Transform (DWT),such as Embedded Zero Wavelet (EZW) which produces excellent compression performance, both in terms of statistical peak signal to noise ratio (PSNR) and subjective human perception of the reconstructed image.

How to interpret wavelet coefficients?

Numerical Analysis of Wavelet Methods Note that the wavelet coefficients have a directional interpretation: the coefficients d j , k a (resp. d j , k b ) indicate the fluctuations in the x (resp. y) direction, i.e. they are particularily sensitive to a vertical (resp. horizontal) edge on the graph of f(x, y).

What is the Zerotree of wavelet coefficients?

The zerotree is based on the hypothesis that if a wavelet coef- ficient at a coarse scale is indocHub with respect to a given threshold T, then all wavelet coefficients of the same orientation in the same spatial location at finer scales are likely to be indocHub with respect to T.

See why our customers choose DocHub

Great solution for PDF docs with very little pre-knowledge required.

"Simplicity, familiarity with the menu and user-friendly. It's easy to navigate, make changes and edit whatever you may need. Because it's used alongside Google, the document is always saved, so you don't have to worry about it."

Pam Driscoll F
Teacher

A Valuable Document Signer for Small Businesses.

"I love that DocHub is incredibly affordable and customizable. It truly does everything I need it to do, without a large price tag like some of its more well known competitors. I am able to send secure documents directly to me clients emails and via in real time when they are viewing and making alterations to a document."

Jiovany A
Small-Business

I can create refillable copies for the templates that I select and then I can publish those.

"I like to work and organize my work in the appropriate way to meet and even exceed the demands that are made daily in the office, so I enjoy working with PDF files, I think they are more professional and versatile, they allow..."

Victoria G
Small-Business

be ready to get more

Edit and sign PDFfor free

Get started now