Italics shape in XPS

Utilize this walkthrough to italics shape in XPS in a snap

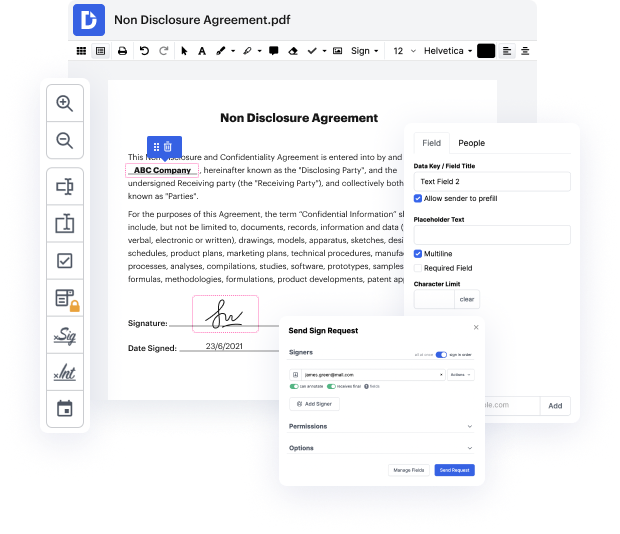
XPS may not always be the simplest with which to work. Even though many editing capabilities are out there, not all provide a easy solution. We designed DocHub to make editing easy, no matter the file format. With DocHub, you can quickly and effortlessly italics shape in XPS. On top of that, DocHub delivers an array of other functionality including form generation, automation and management, industry-compliant eSignature tools, and integrations.

DocHub also helps you save time by creating form templates from paperwork that you use frequently. On top of that, you can make the most of our a wide range of integrations that enable you to connect our editor to your most used apps with ease. Such a solution makes it quick and easy to work with your files without any delays.

To italics shape in XPS, follow these steps:

Hit Log In or register a free account.
When directed to your Dashboard, click the Add New button and select how you want to add your file.
Use our advanced features that will let you enhance your document's content and layout.
Pick the ability to italics shape in XPS from the toolbar and use it on form.
Go over your content once more to make sure it has no errors or typos.
Hit DONE to complete working on your form.

DocHub is a helpful tool for personal and corporate use. Not only does it provide a all-purpose set of features for form generation and editing, and eSignature integration, but it also has an array of capabilities that come in handy for producing multi-level and simple workflows. Anything added to our editor is stored risk-free in accordance with leading industry criteria that safeguard users' information.

Make DocHub your go-to choice and simplify your form-based workflows with ease!

PDF editing simplified with DocHub

Seamless PDF editing

Editing a PDF is as simple as working in a Word document. You can add text, drawings, highlights, and redact or annotate your document without affecting its quality. No rasterized text or removed fields. Use an online PDF editor to get your perfect document in minutes.

Smooth teamwork

Collaborate on documents with your team using a desktop or mobile device. Let others view, edit, comment on, and sign your documents online. You can also make your form public and share its URL anywhere.

Automatic saving

Every change you make in a document is automatically saved to the cloud and synchronized across all devices in real-time. No need to send new versions of a document or worry about losing information.

Google integrations

DocHub integrates with Google Workspace so you can import, edit, and sign your documents directly from your Gmail, Google Drive, and Dropbox. When finished, export documents to Google Drive or import your Google Address Book and share the document with your contacts.

Powerful PDF tools on your mobile device

Keep your work flowing even when you're away from your computer. DocHub works on mobile just as easily as it does on desktop. Edit, annotate, and sign documents from the convenience of your smartphone or tablet. No need to install the app.

Secure document sharing and storage

Instantly share, email, and fax documents in a secure and compliant way. Set a password, place your documents in encrypted folders, and enable recipient authentication to control who accesses your documents. When completed, keep your documents secure in the cloud.

Drive efficiency with the DocHub add-on for Google Workspace

Access documents and edit, sign, and share them straight from your favorite Google Apps.

Install now

How to italics shape in XPS

4.8 out of 5

34 votes

the objective of this video is to illustrate how a background and a combination of two synthetic piece can be used to approximate these data the basic components of a peak model are a background which is defined on the regions property page and synthetic components that are defined on the components property page both on the quantification parameters dialog window the background is added and represents the curve above which signal is considered to be docHub to XPS so once weamp;#39;ve established a background the next thing is to approximate the signal by using synthetic components the objective for pulse counted data is to produce a residual standard deviation that is close to unity so to achieve this we need to adjust the background and also line shapes so that we obtain a good approximation to the signal itself so that when we perform optimization the residual can fit the data as accurately as possible so in the first instance what weamp;#39;ll do is adjust the line shapes an

Got questions?

Below are some common questions from our customers that may provide you with the answer you're looking for. If you can't find an answer to your question, please don't hesitate to reach out to us.

Contact us

Is it XPS or ESCA?

X-Ray Photoelectron Spectroscopy (XPS) is also known as Electron Spectroscopy for Chemical Analysis (ESCA). X-Ray Photoelectron Spectroscopy is used to determine quantitative atomic composition and chemistry. X-ray Photoelectron Spectroscopy | XPS-ESCA - EAG Laboratories EAG Laboratories techniques x-ray-photoelectron EAG Laboratories techniques x-ray-photoelectron

What is the XPS analysis technique?

X-ray Photoelectron Spectroscopy (XPS) or Electron Spectroscopy for Chemical Analysis (ESCA) is a technique which analyzes the elements constituting the sample surface, its composition, and chemical bonding state by irradiating x-rays on the sample surface, and measuring the kinetic energy of the photoelectrons emitted

What are the limitations of XPS spectroscopy?

Because XPS is a surface technique, there is a limited amount of organic information XPS can provide. XPS is limited to measurements of elements having atomic numbers of 3 or greater, making it unable to detect hydrogen or helium. XPS spectra also take a long time to obtain. Applications of Photoelectron Spectroscopy - Chemistry LibreTexts Chemistry LibreTexts Bookshelves Applications Chemistry LibreTexts Bookshelves Applications

What is the chemical shift in XPS?

Chemical shift arises in the initial state from the displacement of the electronic charge from the atom towards its ligands, reducing the electrostatic potential at the atom. There is a final state shift due to the polarization of the ligand by the core on the central atom. X-Ray Photoelectron Spectroscopy (XPS)-2 - Washington State University Washington State University documents 571-XPS-Lecture2001 Washington State University documents 571-XPS-Lecture2001

What is the XPS characterization technique?

X-ray Photoelectron Spectroscopy (XPS) or Electron Spectroscopy for Chemical Analysis (ESCA) is a technique which analyzes the elements constituting the sample surface, its composition, and chemical bonding state by irradiating x-rays on the sample surface, and measuring the kinetic energy of the photoelectrons emitted Techniques:What is XPS? l ULVAC-PHI, Inc. Ulvac PHI surface-analysis xps Ulvac PHI surface-analysis xps

What is the XPS test method?

X-Ray Photoelectron Spectroscopy is used to determine quantitative atomic composition and chemistry. It is a surface analysis technique with a sampling volume that extends from the surface to a depth of approximately 50-100 . XPS Spectroscopy can also be used for sputter depth profiling.

What is characterization of XPS?

XPS is used to characterize the surfaces of diverse materials such as inorganic compounds (minerals), semiconductors, organic compounds, and thin films and coatings on natural and engineered materials.

How does the XPS technique work?

How XPS works. X-rays (photons) are shot onto a sample, and when electrons in the sample absorb enough energy, they are ejected from the sample with a certain kinetic energy. The energy of those ejected electrons is analyzed by a detector and a plot of these energies and relative numbers of electrons is produced.

See why our customers choose DocHub

Great solution for PDF docs with very little pre-knowledge required.

"Simplicity, familiarity with the menu and user-friendly. It's easy to navigate, make changes and edit whatever you may need. Because it's used alongside Google, the document is always saved, so you don't have to worry about it."

Pam Driscoll F
Teacher

A Valuable Document Signer for Small Businesses.

"I love that DocHub is incredibly affordable and customizable. It truly does everything I need it to do, without a large price tag like some of its more well known competitors. I am able to send secure documents directly to me clients emails and via in real time when they are viewing and making alterations to a document."

Jiovany A
Small-Business

I can create refillable copies for the templates that I select and then I can publish those.

"I like to work and organize my work in the appropriate way to meet and even exceed the demands that are made daily in the office, so I enjoy working with PDF files, I think they are more professional and versatile, they allow..."

Victoria G
Small-Business

be ready to get more

Edit and sign PDFfor free

Get started now