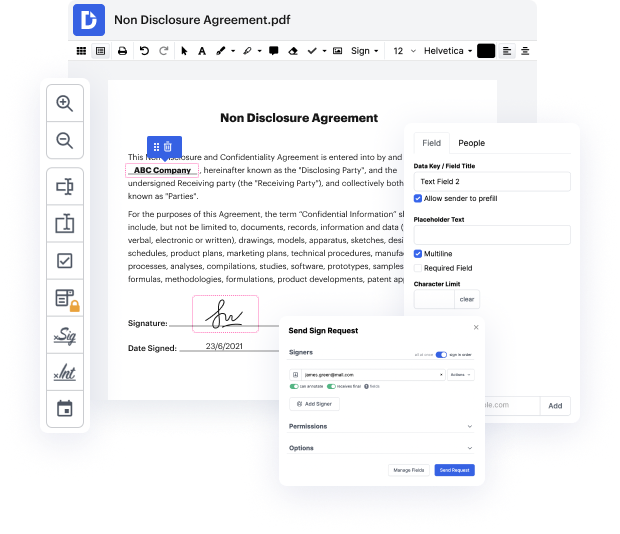
Disadvantages are present in every tool for editing every document type, and although you can find a wide variety of tools on the market, not all of them will fit your particular needs. DocHub makes it easier than ever to make and modify, and deal with paperwork - and not just in PDF format.
Every time you need to easily fill in point in LOG, DocHub has got you covered. You can effortlessly modify form elements including text and images, and layout. Customize, organize, and encrypt paperwork, develop eSignature workflows, make fillable documents for intuitive information gathering, and more. Our templates option allows you to create templates based on paperwork with which you often work.
In addition, you can stay connected to your go-to productivity capabilities and CRM platforms while managing your paperwork.
One of the most incredible things about utilizing DocHub is the option to handle form tasks of any difficulty, regardless of whether you require a fast edit or more diligent editing. It includes an all-in-one form editor, website form builder, and workflow-centered capabilities. In addition, you can be certain that your paperwork will be legally binding and comply with all safety frameworks.
Cut some time off your projects with the help of DocHub's tools that make handling paperwork straightforward.
now letamp;#39;s talk about graphing logarithmic functions letamp;#39;s go over the four basic shapes so letamp;#39;s say if you have log x where x and y are both positive kind of like what we did in the last lesson in this case the graph is going to travel towards quadrant one logarithmic functions are basically the inverse of an exponential function exponential functions contain a horizontal asymptote logarithmic functions contain a vertical asymptote exponential functions they increase at an increase in rate logarithmic functions they increase at a decrease in rate so letamp;#39;s say if we were to put 2 to the x and log to the x on the same graph this is the basic curve of 2 to the x and the graph log base 2 dx looks like this iamp;#39;m going to highlight it in blue these two functions are inverse functions an inverse function reflects across the line y equals zero with its counterpart so letamp;#39;s say this is f this is the inverse of f they reflect about the line y equal