Not all formats, including 1ST, are designed to be effortlessly edited. Even though a lot of features can help us edit all document formats, no one has yet created an actual all-size-fits-all solution.
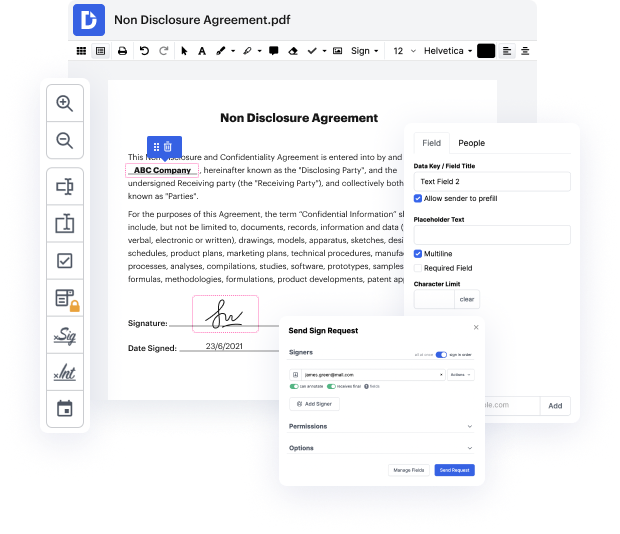
DocHub gives a easy and streamlined solution for editing, taking care of, and storing papers in the most popular formats. You don't have to be a technology-knowledgeable person to embed TIN in 1ST or make other tweaks. DocHub is powerful enough to make the process simple for everyone.
Our feature enables you to change and tweak papers, send data back and forth, create interactive forms for data gathering, encrypt and protect paperwork, and set up eSignature workflows. Additionally, you can also create templates from papers you use regularly.
You’ll find a great deal of other features inside DocHub, including integrations that allow you to link your 1ST document to various productivity applications.
DocHub is a simple, fairly priced option to manage papers and improve workflows. It provides a wide array of tools, from creation to editing, eSignature providers, and web form developing. The software can export your documents in many formats while maintaining highest security and adhering to the greatest data safety requirements.
Give DocHub a go and see just how simple your editing process can be.
if you open any history book on mathematics it will tell you that the ancient Greeks discovered trigonometry our research into this Babylonian tablet is going to change all of that Plimpton three-to-two is a three thousand seven hundred year old fragment of clay from the old babylonian period and itamp;#39;s a scientific marvel of the ancient world weamp;#39;ve known for decades that itamp;#39;s unusual sequence of numbers proves that the Babylonians knew the Pythagorean theorem a thousand years before Pythagoras was born but while there is agreement on what the tablet contains thereamp;#39;s been no agreement on what it was used for our research shows that itamp;#39;s a trigonometric table so unfamiliar and advanced that in some respects itamp;#39;s superior even to modern trigonometry weamp;#39;ve discovered that these lines represent the ratios for a series of right-angled triangles ranging from almost a square to almost a flat line this makes Plimpton three to two a powerful