Not all formats, such as QUOX, are designed to be quickly edited. Even though numerous tools will let us tweak all document formats, no one has yet created an actual all-size-fits-all solution.
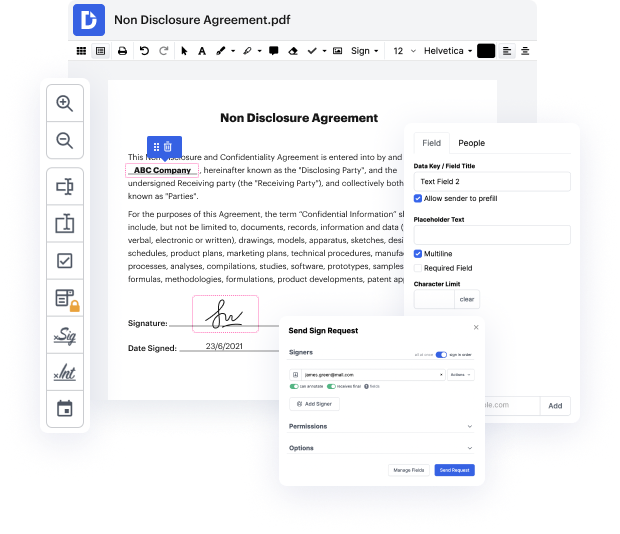
DocHub gives a straightforward and streamlined solution for editing, taking care of, and storing papers in the most widely used formats. You don't have to be a tech-savvy user to darken symbol in QUOX or make other changes. DocHub is robust enough to make the process simple for everyone.
Our tool allows you to modify and edit papers, send data back and forth, generate interactive documents for data collection, encrypt and protect paperwork, and set up eSignature workflows. Moreover, you can also create templates from papers you use on a regular basis.
You’ll locate a great deal of other features inside DocHub, including integrations that let you link your QUOX document to a variety business applications.
DocHub is an intuitive, cost-effective way to manage papers and streamline workflows. It provides a wide selection of capabilities, from creation to editing, eSignature professional services, and web document creating. The application can export your paperwork in many formats while maintaining greatest protection and adhering to the highest data safety criteria.
Give DocHub a go and see just how simple your editing operation can be.
the mathematical symbol of today is the product symbol written with a capital pi and in the same way as we have it for the sum symbol we use an index below the letter Pi so here K starts with the value 1 goes through all natural numbers until we docHub the value n in the end hence the symbol here is a short notation for a product with exactly n factors more precisely we start with a 1 times a 2 times a 3 and so on until we docHub a n of course A1 A2 and so on could be any numbers the important thing is only that we have an index involved on the left hand side we call it K but of course any other name would do it as well now you can see the whole thing here as an explanation for the product symbol but the form of definition should be given by a recursive definition this means that we first have to explain what the empty product should be in other words what happens when we set n to 0. then the explanation from above does not make any sense because there are no numbers involved indeed the