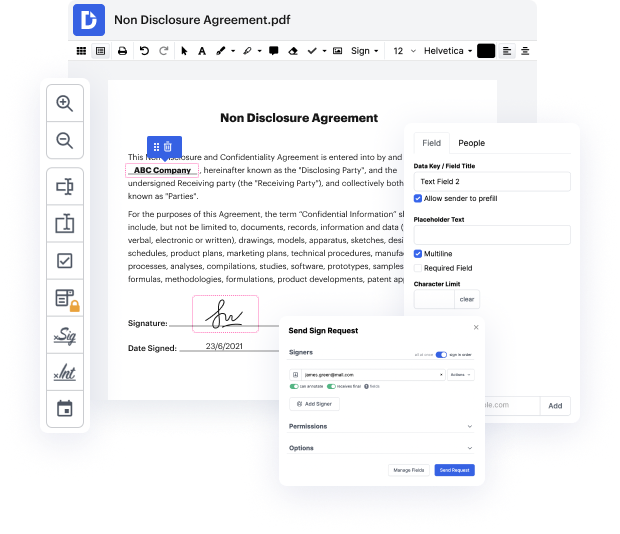
Editing FDX is fast and straightforward using DocHub. Skip downloading software to your computer and make changes using our drag and drop document editor in just a few easy steps. DocHub is more than just a PDF editor. Users praise it for its convenience and powerful capabilities that you can use on desktop and mobile devices. You can annotate documents, generate fillable forms, use eSignatures, and deliver documents for completion to other people. All of this, combined with a competitive price, makes DocHub the ideal option to darken point in FDX files effortlessly.
Make your next tasks even easier by turning your documents into reusable web templates. Don't worry about the security of your information, as we securely keep them in the DocHub cloud.
hi everyone Iamp;#39;m Parker Glen 80 and Iamp;#39;m going to talk about from colorings to fixed points so how we can use colorings of graphs to get fixed points in real analysis okay so this is a proof that could come up in a lot of different courses it could come up in C 32 which is graph theory and algorithms it could come up in C 27 which is topology or if you come up and see 44 were just coming to torques so it sort of could happen in a lot of different places what I like about this proof is that it gives us an algorithm so letamp;#39;s get into it okay so consider a string of beads okay so weamp;#39;re gonna color our beads red and blue okay and theyamp;#39;re going to impose that the first bead has to be blue and the other bead the last bead has to be red so weamp;#39;re going to call such a collection of beads well colored if the first feed is blue and the last bead is ready okay so now I want to draw your attention to a fact about well colored strings of beads if we look