Cut off pecularity in binary

Use this walkthrough to cut off pecularity in binary in a snap

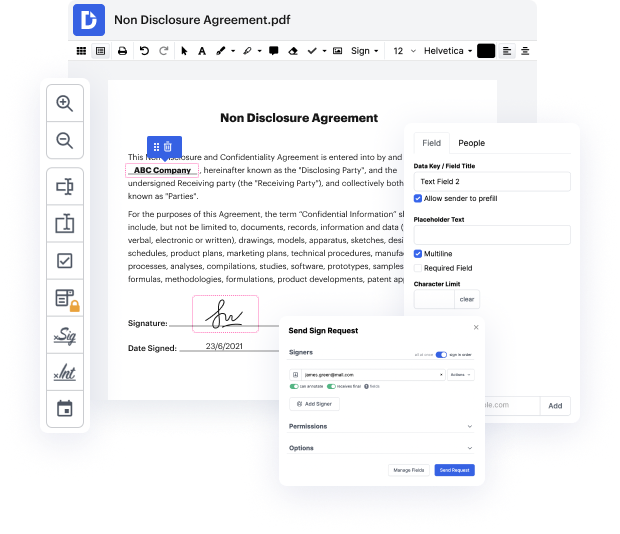
binary may not always be the best with which to work. Even though many editing tools are available on the market, not all offer a simple tool. We developed DocHub to make editing straightforward, no matter the file format. With DocHub, you can quickly and easily cut off pecularity in binary. In addition to that, DocHub provides a variety of additional tools such as document creation, automation and management, industry-compliant eSignature solutions, and integrations.

DocHub also lets you save effort by creating document templates from documents that you utilize regularly. In addition to that, you can make the most of our a lot of integrations that enable you to connect our editor to your most used apps easily. Such a tool makes it fast and simple to deal with your documents without any slowdowns.

To cut off pecularity in binary, follow these steps:

Click Log In or register a free account.
When directed to your Dashboard, click the Add New button and choose how you want to add your file.
Use our pro tools that can help you improve your document's content and design.
Pick the ability to cut off pecularity in binary from the toolbar and use it on document.
Review your content once again to ensure it has no errors or typos.
Click DONE to finish editing document.

DocHub is a handy feature for personal and corporate use. Not only does it offer a all-purpose suite of tools for document creation and editing, and eSignature implementation, but it also has a variety of tools that come in handy for producing multi-level and streamlined workflows. Anything added to our editor is stored risk-free in accordance with major field criteria that safeguard users' data.

Make DocHub your go-to choice and simplify your document-centered workflows easily!

PDF editing simplified with DocHub

Seamless PDF editing

Editing a PDF is as simple as working in a Word document. You can add text, drawings, highlights, and redact or annotate your document without affecting its quality. No rasterized text or removed fields. Use an online PDF editor to get your perfect document in minutes.

Smooth teamwork

Collaborate on documents with your team using a desktop or mobile device. Let others view, edit, comment on, and sign your documents online. You can also make your form public and share its URL anywhere.

Automatic saving

Every change you make in a document is automatically saved to the cloud and synchronized across all devices in real-time. No need to send new versions of a document or worry about losing information.

Google integrations

DocHub integrates with Google Workspace so you can import, edit, and sign your documents directly from your Gmail, Google Drive, and Dropbox. When finished, export documents to Google Drive or import your Google Address Book and share the document with your contacts.

Powerful PDF tools on your mobile device

Keep your work flowing even when you're away from your computer. DocHub works on mobile just as easily as it does on desktop. Edit, annotate, and sign documents from the convenience of your smartphone or tablet. No need to install the app.

Secure document sharing and storage

Instantly share, email, and fax documents in a secure and compliant way. Set a password, place your documents in encrypted folders, and enable recipient authentication to control who accesses your documents. When completed, keep your documents secure in the cloud.

Drive efficiency with the DocHub add-on for Google Workspace

Access documents and edit, sign, and share them straight from your favorite Google Apps.

Install now

How to cut off pecularity in binary

4.8 out of 5

38 votes

hi everyone today we will talk about the decimal and binary number systems and i will of course show you how to convert numbers from one to the other so first letamp;#39;s talk about the decimal system in the decimal system we use digits from 0 to 9 to create numbers and since we are using 10 digits we also call this system base 10 so when we take the number 1024 for example we can see that itamp;#39;s made out of four ones two tens zero hundredths and one thousand and when we look a bit deeper we notice that all the multiplicands are actually powers of 10 because 1 is also 10 in a power of 0. 10 is also 10 in a power of 1. then 100 equals to 10 and a power of 2 and lastly 1000 is 10 in a power of 3. now i am sure that most of us are very familiar with the decimal system from childhood but how about the binary one the binary system is only using two digits to create numbers zero and one and since we only have two digits in our toolbox we call this system base 2. now in base 10 we hav

Got questions?

Below are some common questions from our customers that may provide you with the answer you're looking for. If you can't find an answer to your question, please don't hesitate to reach out to us.

Contact us

What is the cutoff for classification model?

The standard cutoff is 0.5, which means that if the predicted probability is greater than 0.5, that observation is classified as a positive (or simply as a 1). Classification methods for multiple logistic regression - GraphPad GraphPad prism latest curve-fitting GraphPad prism latest curve-fitting

What is cut off binary classification?

Imagine a binary classification problem. Lets say I have 800,000 predicted probabilities stored in predtest . I define a cutoff as any value in predtest such that the values that are greater than or equal to cutoff are assigned the value 1 and the values that are smaller than cutoff are assigned the value 0.

What does off mean in binary?

Since the binary system uses only two digits or bits and represents numbers using varying patterns of 1s and 0s, it is known as a base-2 system. Here, 1 refers to on or true, while 0 refers to off or false.

What is the cutoff value in data mining?

Typically, over-all-error rate is lowest when the cutoff is 0.50, so this is the default cutoff rate in data mining tools. This makes sense if the cost of both types of errors have approximately equal costs. 9.3 Cutoff Rate and Probability Table - MyEducator MyEducator reader web MyEducator reader web

How to decide cut-off in logistic regression?

Your cutoff point depends on the relative cost of a false positive and a false negative, and what you want their ratio to be (which depends on the predicted probability, and the base rate). If youre looking for cancers, you want that cutoff to be low, because you dont want to miss any cancers. How to estimate the cutoff point in logistic regression - Quora Quora How-can-I-estimate-the-cutoff- Quora How-can-I-estimate-the-cutoff-

What is the cutoff for binary logistic regression?

The contingency table used here computes predicted probabilities based on the model and then classifies the probabilities using a cut-off of 0.5. So, any predicted probability of 0.5 or greater is classified as having the outcome and any predicted probability below 0.5 is classified as not having the outcome. Primer on binary logistic regression - PMC - NCBI NCBI articles PMC8710907 NCBI articles PMC8710907

See why our customers choose DocHub

Great solution for PDF docs with very little pre-knowledge required.

"Simplicity, familiarity with the menu and user-friendly. It's easy to navigate, make changes and edit whatever you may need. Because it's used alongside Google, the document is always saved, so you don't have to worry about it."

Pam Driscoll F
Teacher

A Valuable Document Signer for Small Businesses.

"I love that DocHub is incredibly affordable and customizable. It truly does everything I need it to do, without a large price tag like some of its more well known competitors. I am able to send secure documents directly to me clients emails and via in real time when they are viewing and making alterations to a document."

Jiovany A
Small-Business

I can create refillable copies for the templates that I select and then I can publish those.

"I like to work and organize my work in the appropriate way to meet and even exceed the demands that are made daily in the office, so I enjoy working with PDF files, I think they are more professional and versatile, they allow..."

Victoria G
Small-Business

be ready to get more

Edit and sign PDFfor free

Get started now