Conceal space in CCF

Conceal space in CCF with our multi-purpose editing solution

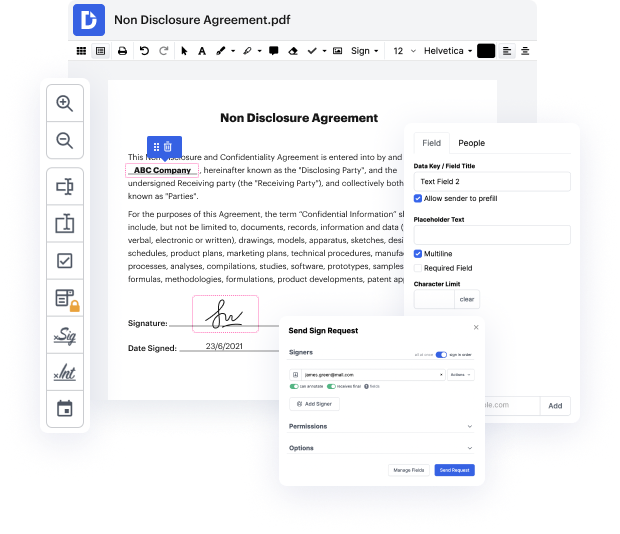
Regardless of how labor-intensive and challenging to modify your files are, DocHub offers a straightforward way to change them. You can modify any element in your CCF without extra resources. Whether you need to modify a single element or the whole form, you can rely on our robust solution for quick and quality outcomes.

In addition, it makes sure that the final file is always ready to use so that you can get on with your tasks without any slowdowns. Our all-encompassing set of features also includes sophisticated productivity tools and a collection of templates, enabling you to make the most of your workflows without the need of wasting time on repetitive activities. On top of that, you can gain access to your papers from any device and integrate DocHub with other solutions.

How to conceal space in CCF

Start by hitting our free trial option or signing in to your existing account.
Upload your form to DocHub’s editor.
Explore DocHub’s tools and find the option to conceal space in CCF.
Check your form for any typos or errors.
Click DONE to use changes. Use any delivery option and other features for organizing your papers.

DocHub can handle any of your form management activities. With a great deal of features, you can generate and export papers however you prefer. Everything you export to DocHub’s editor will be stored securely for as long as you need, with strict safety and information protection protocols in place.

Check DocHub now and make managing your files simpler!

PDF editing simplified with DocHub

Seamless PDF editing

Editing a PDF is as simple as working in a Word document. You can add text, drawings, highlights, and redact or annotate your document without affecting its quality. No rasterized text or removed fields. Use an online PDF editor to get your perfect document in minutes.

Smooth teamwork

Collaborate on documents with your team using a desktop or mobile device. Let others view, edit, comment on, and sign your documents online. You can also make your form public and share its URL anywhere.

Automatic saving

Every change you make in a document is automatically saved to the cloud and synchronized across all devices in real-time. No need to send new versions of a document or worry about losing information.

Google integrations

DocHub integrates with Google Workspace so you can import, edit, and sign your documents directly from your Gmail, Google Drive, and Dropbox. When finished, export documents to Google Drive or import your Google Address Book and share the document with your contacts.

Powerful PDF tools on your mobile device

Keep your work flowing even when you're away from your computer. DocHub works on mobile just as easily as it does on desktop. Edit, annotate, and sign documents from the convenience of your smartphone or tablet. No need to install the app.

Secure document sharing and storage

Instantly share, email, and fax documents in a secure and compliant way. Set a password, place your documents in encrypted folders, and enable recipient authentication to control who accesses your documents. When completed, keep your documents secure in the cloud.

Drive efficiency with the DocHub add-on for Google Workspace

Access documents and edit, sign, and share them straight from your favorite Google Apps.

Install now

How to conceal space in CCF

5 out of 5

20 votes

hello everyone in this session we will discuss a topic that is ccf and the ocf so what is ccf ccf means controllable canonical form controllable canonical form so what is this meaning so up to now we discussed some problems that is regarding the state model the equations are x dot equal to ax plus bu and y equals cx plus du these equations is called as the controllable canonical form and we can and this ccf controllable canonical form can be converted into the ocf that is observable canonical form so both this controllable and observable canonical form both are similar together but it has a small modification that is this is also this will also represent the same equation x dot equal to ax plus bu and y equal to cx plus du these are also the same equations but we have a small difference this a is this a is i will write controllable canonical form a okay this b is controllable canonical form b this c is controllable canonical form this d is controllable canonical form here a b c

Got questions?

Below are some common questions from our customers that may provide you with the answer you're looking for. If you can't find an answer to your question, please don't hesitate to reach out to us.

Contact us

What is the Jordan form of state space?

In linear algebra, a Jordan normal form, also known as a Jordan canonical form, is an upper triangular matrix of a particular form called a Jordan matrix representing a linear operator on a finite-dimensional vector space with respect to some basis.

What is observable canonical form state space?

Observable Canonical Form In this form, the characteristic polynomial of the system appears explicitly in the last column of the A matrix. Observable canonical form can be obtained from the controllable canonical form as follows: A o b s = A c o n t T B o b s = C c o n t T C o b s = B c o n t T D o b s = D c o n t T .

What is state space in advance control system?

 State-space notation is a set of matrix equations which describe how a system will evolve over time. These equations relate the change in state , and the output , to linear combinations of the current state vector and input vector . State-space control can deal with continuous-time and discrete-time systems.

What is the procedure for Jordan form?

To find the Jordan form carry out the following procedure for each eigen- value of A. First solve (A I)v = 0, counting the number r1 of lin- early independent solutions. If r1 = r good, otherwise r1 r and we must now solve (A I)2v = 0. There will be r2 linearly independent solu- tions where r2 r1.

What is state space representation in control system problems?

State space representation is a mathematical model of a physical system expressed as a function of input, output, and state variables related by first-order differential equations or difference equations.

What is Jordan form of system?

A matrix is said to be in Jordan form if 1) its diagonal entries are equal to its eigenvalues; 2) its supradiagonal entries are either zeros or ones; 3) all its other entries are zeros.

What are the different forms of state space?

Four state space forms the phase variable form (controller form), the observer form, the modal form, and the Jordan form-which are often used in modern control theory and practice, are presented.

See why our customers choose DocHub

Great solution for PDF docs with very little pre-knowledge required.

"Simplicity, familiarity with the menu and user-friendly. It's easy to navigate, make changes and edit whatever you may need. Because it's used alongside Google, the document is always saved, so you don't have to worry about it."

Pam Driscoll F
Teacher

A Valuable Document Signer for Small Businesses.

"I love that DocHub is incredibly affordable and customizable. It truly does everything I need it to do, without a large price tag like some of its more well known competitors. I am able to send secure documents directly to me clients emails and via in real time when they are viewing and making alterations to a document."

Jiovany A
Small-Business

I can create refillable copies for the templates that I select and then I can publish those.

"I like to work and organize my work in the appropriate way to meet and even exceed the demands that are made daily in the office, so I enjoy working with PDF files, I think they are more professional and versatile, they allow..."

Victoria G
Small-Business

be ready to get more

Edit and sign PDFfor free

Get started now

Conceal space in CCF with our multi-purpose editing solution

How to conceal space in CCF

PDF editing simplified with DocHub

Drive efficiency with the DocHub add-on for Google Workspace

How to conceal space in CCF

Got questions?

What is the Jordan form of state space?

What is observable canonical form state space?

What is state space in advance control system?

What is the procedure for Jordan form?

What is state space representation in control system problems?

What is Jordan form of system?

What are the different forms of state space?

See why our customers choose DocHub

Edit and sign PDFfor free

Try more PDF tools