Blot out line in jpeg

Use this walkthrough to blot out line in jpeg quickly

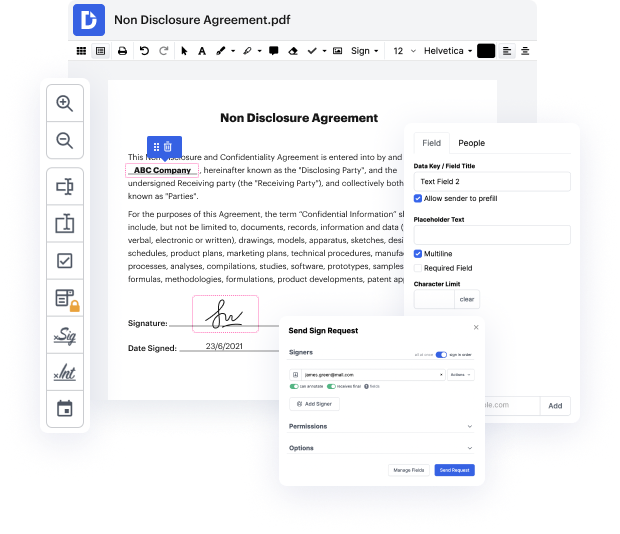
jpeg may not always be the easiest with which to work. Even though many editing features are out there, not all give a easy solution. We designed DocHub to make editing easy, no matter the file format. With DocHub, you can quickly and easily blot out line in jpeg. In addition to that, DocHub offers a range of additional tools such as document creation, automation and management, industry-compliant eSignature solutions, and integrations.

DocHub also allows you to save effort by creating document templates from paperwork that you utilize frequently. In addition to that, you can take advantage of our numerous integrations that allow you to connect our editor to your most utilized apps easily. Such a solution makes it quick and easy to deal with your files without any delays.

To blot out line in jpeg, follow these steps:

Click Log In or create a free account.
When forwarded to your Dashboard, click the Add New button and select how you want to add your file.
Use our sophisticated tools that can help you improve your document's text and layout.
Select the ability to blot out line in jpeg from the toolbar and apply it to document.
Review your text once more to make sure it has no mistakes or typos.
Click DONE to complete editing document.

DocHub is a useful feature for personal and corporate use. Not only does it give a all-encompassing collection of tools for document creation and editing, and eSignature integration, but it also has a range of features that prove useful for developing complex and streamlined workflows. Anything added to our editor is stored safe in accordance with leading industry criteria that protect users' data.

Make DocHub your go-to option and streamline your document-based workflows easily!

PDF editing simplified with DocHub

Seamless PDF editing

Editing a PDF is as simple as working in a Word document. You can add text, drawings, highlights, and redact or annotate your document without affecting its quality. No rasterized text or removed fields. Use an online PDF editor to get your perfect document in minutes.

Smooth teamwork

Collaborate on documents with your team using a desktop or mobile device. Let others view, edit, comment on, and sign your documents online. You can also make your form public and share its URL anywhere.

Automatic saving

Every change you make in a document is automatically saved to the cloud and synchronized across all devices in real-time. No need to send new versions of a document or worry about losing information.

Google integrations

DocHub integrates with Google Workspace so you can import, edit, and sign your documents directly from your Gmail, Google Drive, and Dropbox. When finished, export documents to Google Drive or import your Google Address Book and share the document with your contacts.

Powerful PDF tools on your mobile device

Keep your work flowing even when you're away from your computer. DocHub works on mobile just as easily as it does on desktop. Edit, annotate, and sign documents from the convenience of your smartphone or tablet. No need to install the app.

Secure document sharing and storage

Instantly share, email, and fax documents in a secure and compliant way. Set a password, place your documents in encrypted folders, and enable recipient authentication to control who accesses your documents. When completed, keep your documents secure in the cloud.

Drive efficiency with the DocHub add-on for Google Workspace

Access documents and edit, sign, and share them straight from your favorite Google Apps.

Install now

How to blot out line in jpeg

4.7 out of 5

39 votes

In the last video, we talked about the beginnings of JPEG, so what do we do at the beginning of the process to start preparing for the discrete cosine transform, which is really how the lossy compression happens within a JPEG. We start with our RGB image, we convert that into YCbCr color space, which separates illuminance and chrominance. And then we can down sample the chrominance if we want, and we can kind of get away with quite a bit of down sampling there that people wonamp;#39;t be able to see. The next step is the discrete cosine transform. Before we start talking about how images are compressed using the discrete cosine transform, itamp;#39;s much better just to start with a simple example of what a discrete cosine transform is and how it works. A cosine function, for anyone who isnamp;#39;t familiar with it, is a function that goes between 1 and -1. What we tend to do on this x-axis is go from 0, to pi, to 2*pi. This is in radians, those of you familiar with degrees, this i

Related features

Fix dent in AWW

Fix dent in CCF

Fix dent in CWK

Fix dent in DBK

Fix dent in DITA

Fix dent in DOCM

Fix dent in DOTX

Fix dent in DWD

Got questions?

Below are some common questions from our customers that may provide you with the answer you're looking for. If you can't find an answer to your question, please don't hesitate to reach out to us.

Contact us

How do I remove an edited line from a photo?

How to Remove Unwanted Object From Photo Eraser Start a new project with signing up. Go to Design. Search for AI Magic tool. Click on Eraser Tool. Adjust Your Brush and remove unwanted passport photo . click on Finish and Download Support images in all formats including JPG, JPEG, PNG, HEIC, WEBP, TIFF.

How do I clarify a JPEG image?

Open your image in the editor, then tap or click on it to open the image editing menu. Select the Adjustments option and then use the Sharpen sliding scale to add clarity to your image.

How do I remove a line from a jpg file?

To remove a line from a photo, you can use photo editing software like Photoshop or online tools such as Pixlr or GIMP. Select a tool like the clone stamp or healing brush to replace the line with nearby pixels, blending it seamlessly into the surrounding area

How to get rid of drawn lines on a photo?

If you are willing to, Fotor- the Best Photo Eraser would be your best choice to remove item from pictures such as scribbles and merely selecting the area and a click of the removal button can provide you with a more stunning photo.

How do I remove edits from edited photos?

On your computer, go to photos.google.com. Open the photo with edits you want to remove. Click Edit . Click Revert Save.

Can you uncompress a JPEG?

These are generally unfixable. In any case, it is highly advised to save the original image before compression to avoid losing the finer details indefinitely.

How do I remove edited text from a picture?

If you want to remove text from images, follow these simple steps. Download PhotoDirector The Best App To Remove Text from Images. Tap AI Removal to Remove Text from the Image. Adjust the Removal Area Manually. Save the Photo with Text Removed and Share It with Others.

See why our customers choose DocHub

Great solution for PDF docs with very little pre-knowledge required.

"Simplicity, familiarity with the menu and user-friendly. It's easy to navigate, make changes and edit whatever you may need. Because it's used alongside Google, the document is always saved, so you don't have to worry about it."

Pam Driscoll F
Teacher

A Valuable Document Signer for Small Businesses.

"I love that DocHub is incredibly affordable and customizable. It truly does everything I need it to do, without a large price tag like some of its more well known competitors. I am able to send secure documents directly to me clients emails and via in real time when they are viewing and making alterations to a document."

Jiovany A
Small-Business

I can create refillable copies for the templates that I select and then I can publish those.

"I like to work and organize my work in the appropriate way to meet and even exceed the demands that are made daily in the office, so I enjoy working with PDF files, I think they are more professional and versatile, they allow..."

Victoria G
Small-Business

be ready to get more

Edit and sign PDFfor free

Get started now