Not all formats, such as EZW, are created to be effortlessly edited. Even though many features will let us edit all document formats, no one has yet invented an actual all-size-fits-all solution.
DocHub provides a straightforward and efficient solution for editing, handling, and storing paperwork in the most widely used formats. You don't have to be a technology-savvy user to blot out formula in EZW or make other modifications. DocHub is powerful enough to make the process straightforward for everyone.
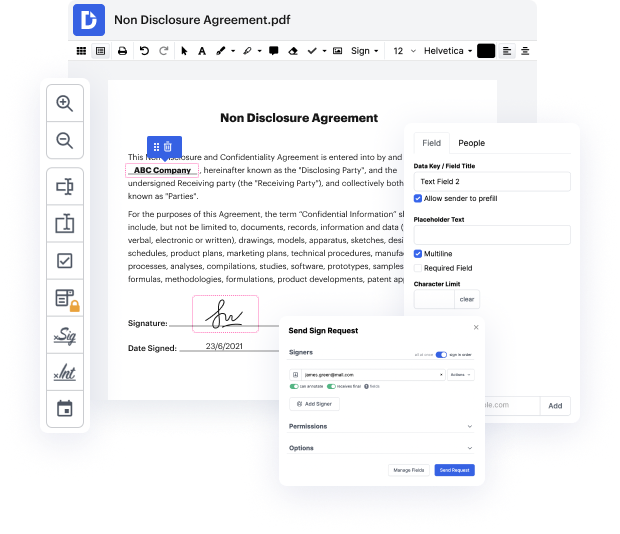
Our feature enables you to change and edit paperwork, send data back and forth, generate interactive forms for data collection, encrypt and safeguard forms, and set up eSignature workflows. Additionally, you can also generate templates from paperwork you use frequently.
You’ll find plenty of other features inside DocHub, such as integrations that allow you to link your EZW document to various business applications.
DocHub is an intuitive, cost-effective option to deal with paperwork and streamline workflows. It offers a wide range of features, from generation to editing, eSignature solutions, and web form creating. The program can export your documents in multiple formats while maintaining highest security and following the maximum data security standards.
Give DocHub a go and see just how straightforward your editing transaction can be.
so here it is connections for connection so weamp;#39;ll take a situation as follows here is the point of X equals a output just a here here is the x-axis and I will imagine that I have a linear potential so we have a linear potential here why do we imagine a linear potential itamp;#39;s because the thing we would really kind of want to think about he said what is called the turning point energy and a potential thereamp;#39;s a turning point but itamp;#39;s clear that near enough to the turning point this is linear and the problem for the wkb approximation fails as we discuss is precisely in this region now I will make one claim that this is really whatamp;#39;s going to connect here these two asymptotic expansions that we found here are in fact wkb approximation of our calculation so our whole procedure here is going to be assuming that we have a linear potential here and I will take the linear potential seriously so I will imagine it even goes forever and what weamp;#39;re goin