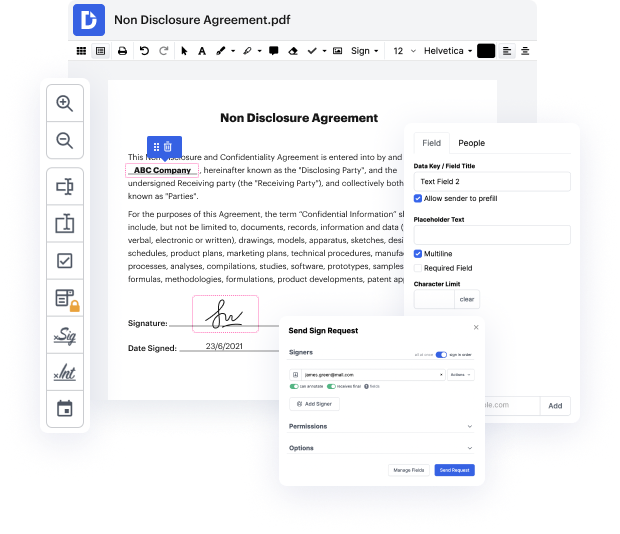
UOML may not always be the best with which to work. Even though many editing features are available on the market, not all provide a simple solution. We created DocHub to make editing effortless, no matter the file format. With DocHub, you can quickly and easily put in point in UOML. In addition to that, DocHub gives a variety of other features such as document creation, automation and management, industry-compliant eSignature tools, and integrations.
DocHub also lets you save time by creating document templates from paperwork that you use frequently. In addition to that, you can take advantage of our a wide range of integrations that allow you to connect our editor to your most utilized applications with ease. Such a solution makes it quick and easy to deal with your documents without any delays.
DocHub is a helpful feature for personal and corporate use. Not only does it provide a comprehensive suite of capabilities for document creation and editing, and eSignature integration, but it also has a variety of features that come in handy for developing multi-level and straightforward workflows. Anything imported to our editor is saved safe according to leading industry standards that protect users' information.
Make DocHub your go-to choice and streamline your document-centered workflows with ease!
in this video weamp;#39;re going to talk about point-slope form a particular form of a linear equation a linear equation can be written in standard form you could write it in slope-intercept form or you can write it in point slope form the formula for linear equation and point-slope form is this y minus y1 is equal to M minus X1 so letamp;#39;s talk about how to use it so letamp;#39;s say we have a graph letamp;#39;s call that the x-axis this is the y-axis and we have a linear equation that looks like this now letamp;#39;s just say just to keep things simple that at this point is that 2 comma five x is 2y is five and letamp;#39;s say this line has a slope of 3. with this information you can write a linear equation in point-slope form our X1 value is 2 and our y1 value is 5. so we can replace y one with five M the slope is 3 and we can replace X1 with two so this is our linear equation in point slope form all we need to write it is the slope and a point which is what we have here