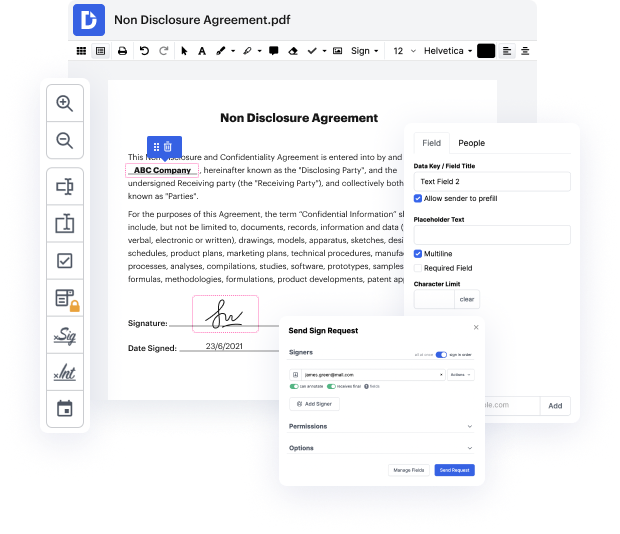
LOG may not always be the easiest with which to work. Even though many editing capabilities are out there, not all give a easy solution. We designed DocHub to make editing effortless, no matter the file format. With DocHub, you can quickly and easily cover up point in LOG. Additionally, DocHub provides an array of additional tools including form creation, automation and management, sector-compliant eSignature services, and integrations.
DocHub also enables you to save effort by creating form templates from paperwork that you use regularly. Additionally, you can benefit from our a wide range of integrations that allow you to connect our editor to your most used apps easily. Such a solution makes it quick and easy to work with your files without any slowdowns.
DocHub is a helpful tool for individual and corporate use. Not only does it give a extensive set of features for form creation and editing, and eSignature implementation, but it also has an array of capabilities that come in handy for producing complex and simple workflows. Anything added to our editor is kept safe according to major industry criteria that shield users' data.
Make DocHub your go-to choice and simplify your form-centered workflows easily!
in this lesson youamp;#39;re gonna learn how to graph logarithmic functions with transformations so the form that weamp;#39;re gonna be working with here is y equals log base B of X minus h plus K so youamp;#39;ve already probably learned how to graph exponential functions theyamp;#39;re graphs that look something like this where there youamp;#39;re either exponential growth or exponential decay but what logarithmic functions are is theyamp;#39;re inverses of exponential functions when you graph an inverse of a function youamp;#39;re reflecting it over this line y equals x so our la graphs are gonna look something like this where theyamp;#39;re going up like slower and slower and the main thing with working with logarithmic graphs is knowing how to switch from the Log form to the exponential form because weamp;#39;re usually not so familiar with thinking in terms of logs so if we can transform it into an exponential form itamp;#39;s a little bit easier to get a graph so weam